
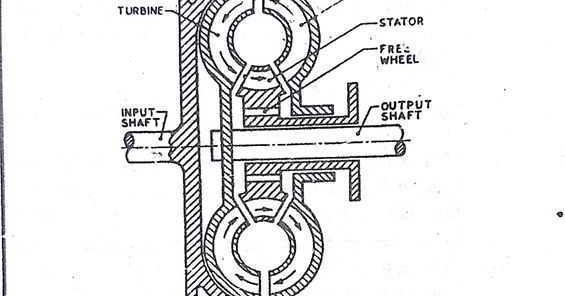
Going off of that, we have angular acceleration (α – lower-case Greek letter alpha) in units of radians per second squared (rad/s 2). In SI The SI unites would be radians per second (rad/s). In Cartesian velocity, we had units of m/s.īecause the axis is rotational for angular velocity, the units are in circular terms. Next is angular velocity (ω – lower-case Greek letter omega). The rotational equivalent would be the angular displacement (θ – lower-case Greek letter theta), and it is in radians (rad). This is necessary because we’re now operating on a different axis and plane.įirst up, we have distance. Other Implications of TorqueĪs a result of torque’s rotational nature, there are certain terms that go along with it that have Cartesian analogs.
Torque diagrams free#
As we will see in our free response example from an AP® Physics test, skillfully choosing the starting point for r can simplify torque problems. The r vector in the torque equation can technically start at any point so long as it ends at the point on the object where the force is applied. It should also be noted that torque can be calculated on different points on an object. In this case, newton-meters and joules are not interchangeable due to their implications. Instead, the units are just newton-meters (N This means that, although it is a force multiplied by a distance, its units aren’t in joules (J) like work or energy. Therefore, torque only occurs if the force applied to the object has a component that is perpendicular to the r vector.

For torque, on the other hand, because of the sine term, there would be no torque if the force were applied parallel or anti parallel to the direction of motion, because sin (0°) = sin (180°) = 0. That means that if the force occurs perpendicular to the direction of motion, the work done would be zero because that would mean our cosine term would be cos (90°) = 0. Because of the cross product, we end up with an equation with a sine term, rather than the dot product for work, which netted us a cosine term. We’ll go over an example later on in this article to show you how you can go ahead and solve one of these.įirst, let’s look at the physical meaning of this equation for one second. This can make torque problems quite tricky and difficult to understand.
Torque is the cross product of two vectors, which means that torque is also a vector. Physically, it is the distance from the axis of rotation to the point where the force is applied. To calculate torque we must introduce a new vector, r. We also can’t use the distance d that we applied in the previous work equation because we are not displacing the object a certain distance. Therefore, instead of a dot product, we need to use a cross product. Torques, however, involve rotation along a fixed axis. Because it is the dot product of two vectors, it is a scalar value. For work, the force ( F) and the distance ( d) were multiplied by a dot product. However, the difference lies in how force and distance are multiplied, and what kind of distance is used in this scenario. Like work, torque is a force multiplied by a distance. The seesaw’s fulcrum fixes it on an axis that causes rotational motion. However, if I take that box and put it on one end of a seesaw, with myself standing at the other, both the box and I are applying torques on the seesaw. If I push it onto an inclined plane to load it onto the back of a truck, it’s still work because the box is not fixed on any particular axis.

If there is a box and I am pushing it horizontally, I am doing work on it. Let’s clarify the difference with examples. Now, what if we take that same object and rotate it around a fixed axis? When we do that, we are applying a torque on that object. If you’re reading about torque, you’ve probably already covered work, which measures a force applied that displaces an object in any direction on a Cartesian coordinate system (x/y coordinates). Introduction to Torque Animated GIF image demonstrating relationship between force (F), torque (τ), linear momentum (p), angular momentum (L), and position (r) of rotating particle.


 0 kommentar(er)
0 kommentar(er)
